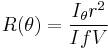Rayleigh ratio

The Rayleigh ratio is a quantity used to characterize the scattered intensity as a function of scattering angle 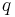q, and is defined as

R( \theta ) = \frac{I_\theta r^2}{I f V}

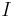where I is the intensity of the incident radiation, 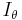I_\theta is the total intensity of scattered radiation observed at an angle 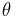\theta and a distance r from the point of scattering and V is the scattering volume. The factor f is introduced to compensate for polarization phenomena, and is dependent of the type of radiation used as follows:

1. For light scattering, f depends on the polarization of the incident beam, and is 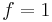f=1 for vertically polarized light, 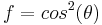f=cos^2 (\theta) for horizontally polarized light and 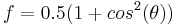f = 0.5(1 %2B cos^2 (\theta)) for unpolarized light.

2. For small-angle neutron scattering, 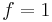f=1.

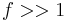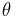3. For small-angle X-ray scattering, f>>1, if \theta < ~ 5° .

Notes:

1. The dimension of 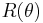R(\theta) is an inverse length.

2. In small-angle neutron scattering the term cross-section is frequently used in place of 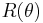R(\theta).

3. IUPAC also recommends the symbol 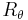R_\theta.

References